Chapter 2: Reference Systems
Chapter Objectives
Upon completion of this chapter you will be able to describe the system of terrestrial coordinates, the rotation of Earth, precession, nutation, and the revolution of Earth about the Sun. You will be able to describe how the locations of celestial objects are stated in the coordinate systems of the celestial sphere. You will be able to describe the use of epochs and various conventions of timekeeping.
Spatial coordinates and timing conventions are adopted in order to consistently identify locations and motions of an observer, of natural objects in the solar system, and of spacecraft traversing interplanetary space or orbiting planets or other bodies. Without these conventions it would be impossible to navigate the solar system.
Terrestrial Coordinates
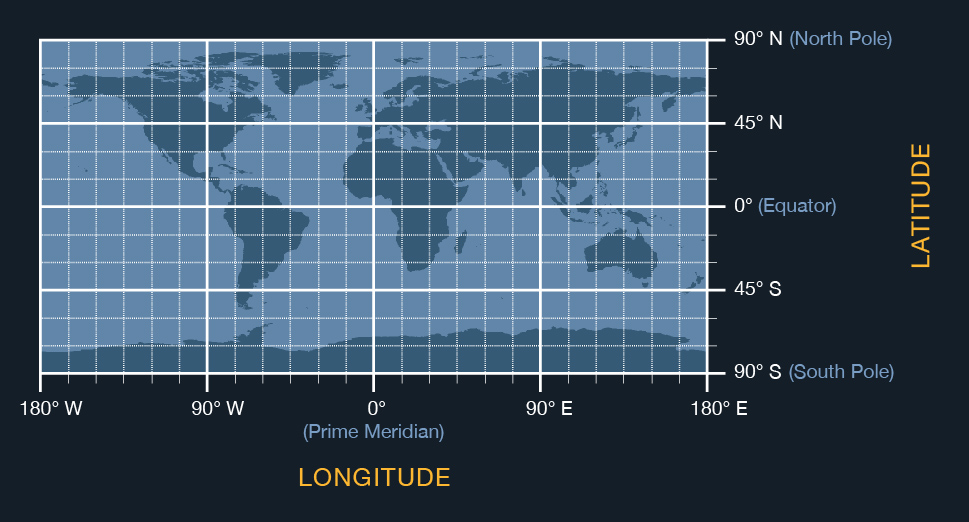
A great circle is an imaginary circle on the surface of a sphere whose center is the center of the sphere. Great circles that pass through both the north and south poles are called meridians, or lines of longitude. For any point on the surface of Earth a meridian can be defined.
The prime meridian, the starting point measuring the east-west locations of other meridians, marks the site of the old Royal Observatory in Greenwich, England. Longitude is expressed in degrees, minutes, and seconds of arc from 0 to 180 degrees eastward or westward from the prime meridian. For example, downtown Pasadena, California, is located at 118 degrees, 8 minutes, 41 seconds of arc west of the prime meridian: 118° 8' 41" W.
The starting point for measuring north-south locations on Earth is the equator, a great circle which is everywhere equidistant from the poles. Circles in planes parallel to the equator define north-south measurements called parallels, or lines of latitude. Latitude is expressed as an arc subtended between the equator and the parallel, as seen from the center of the Earth. Downtown Pasadena is located at 34 degrees, 08 minutes, 44 seconds latitude north of the equator: 34° 08' 44" N.
Throughout the history of navigation, determining one's latitude on the Earth's surface has been relatively easy. In the northern hemisphere for example, simply measuring the height of the star Polaris above the horizon results in a fairly close approximation of one's latitude. Measurement of longitude, however, has been a historically siginificant endeavor, since its determination requires accurate timekeeping. John Harrison (1693-1776) eventually succeeded in developing a chronometer good enough to do the trick.
One degree of latitude equals approximately 111 km on the Earth's surface, and by definition exactly 60 nautical miles. Because meridians converge at the poles, the length of a degree of longitude varies from 111 km at the equator to 0 at the poles where longitude becomes a point.
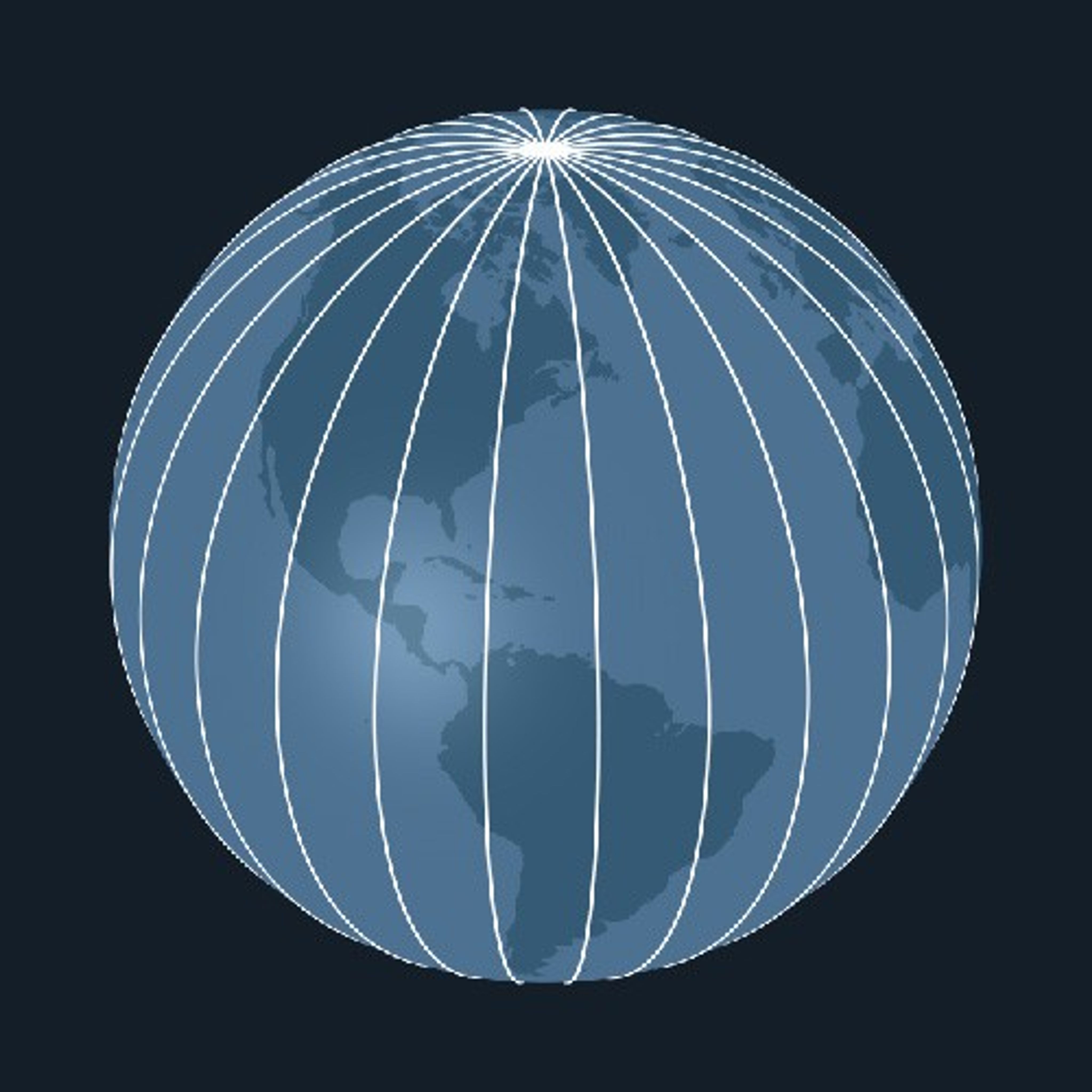
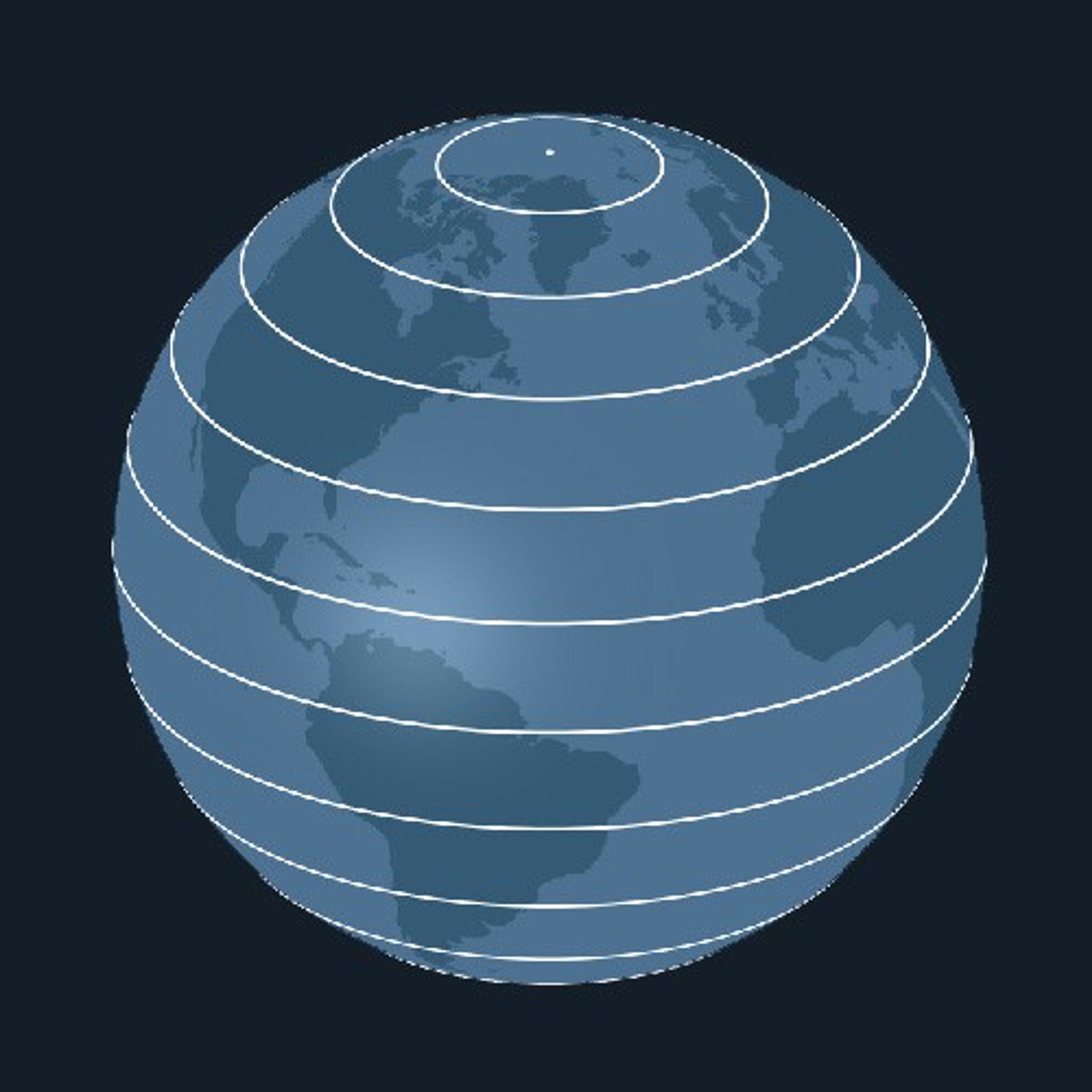
Terrestrial Coordinates Grid
Rotation and Revolution
"Rotation" refers to an object's spinning motion about its own axis. "Revolution" refers the object's orbital motion around another object. For example, Earth rotates on its own axis, producing the 24-hour day. Earth revolves about the Sun, producing the 365-day year. A satellite revolves around a planet.
Earth's Rotation
The Earth rotates on its axis relative to the Sun every 24.0 hours mean solar time, with an inclination of 23.45 degrees from the plane of its orbit around the Sun. Mean solar time represents an average of the variations caused by Earth's non-circular orbit. Its rotation relative to "fixed" stars (sidereal time) is 3 minutes 56.55 seconds shorter than the mean solar day, the equivalent of one solar day per year.
Precession of Earth's Axis Over 26,000 Years
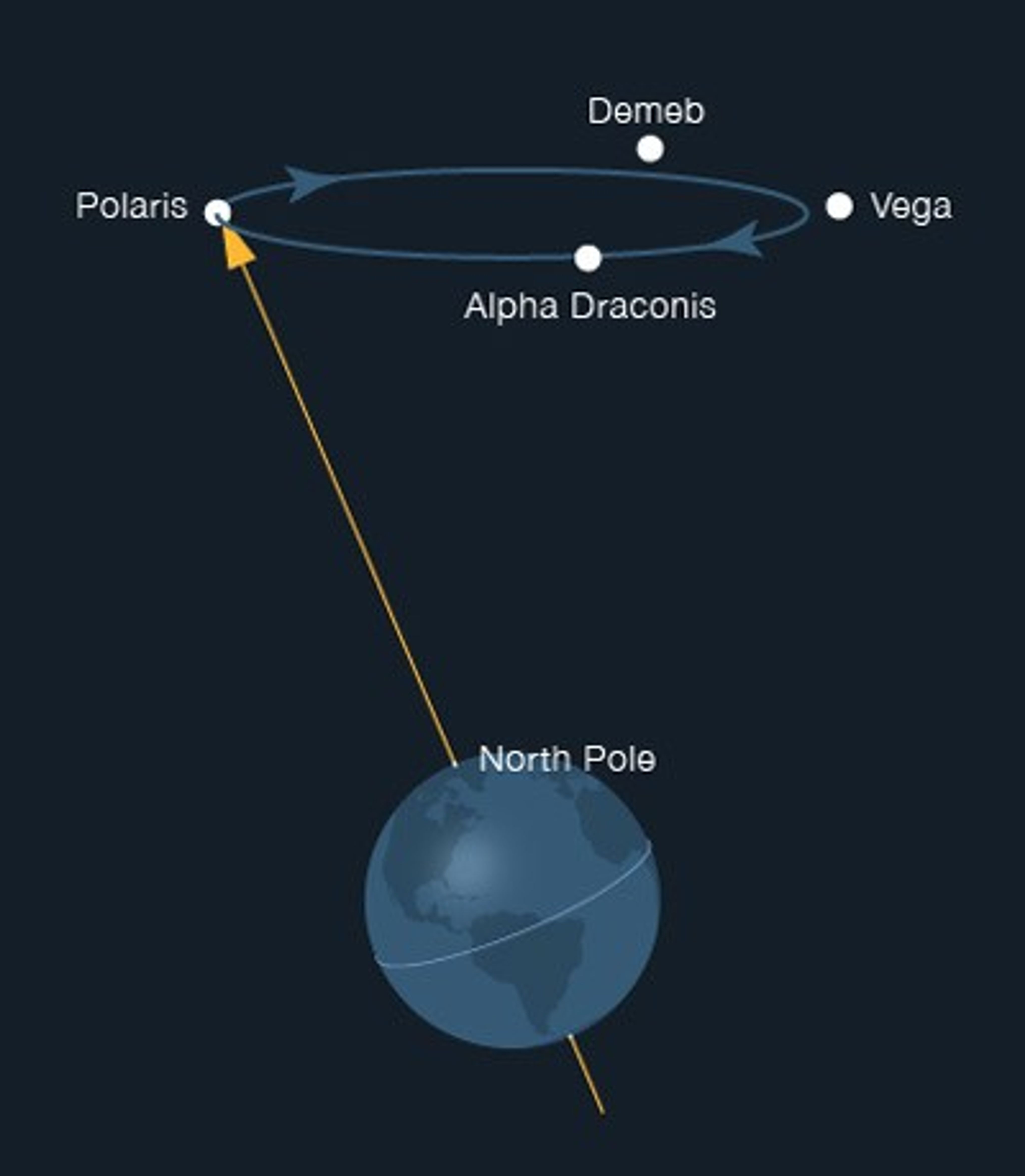
Forces associated with the rotation of Earth cause the planet to be slightly oblate, displaying a bulge at the equator. The moon's gravity primarily, and to a lesser degree the Sun's gravity, act on Earth's oblateness to move the axis perpendicular to the plane of Earth's orbit. However, due to gyroscopic action, Earth's poles do not "right themselves" to a position perpendicular to the orbital plane. Instead, they precess at 90 degrees to the force applied. This precession causes the axis of Earth to describe a circle having a 23.4 degree radius relative to a fixed point in space over about 26,000 years, a slow wobble reminiscent of the axis of a spinning top swinging around before it falls over.
Because of the precession of the poles over 26,000 years, all the stars, and other celestial objects, appear to shift west to east at the rate of .014 degree each year (360 degrees in 26,000 years). This apparent motion is the main reason for astronomers as well as spacecraft operators to refer to a common epoch such as J2000.0.
At the present time in Earth's 26,000 year precession cycle, a bright star happens to be very close, less than a degree, from the north celestial pole. This star is called Polaris, or the North Star.
Stars do have their own real motion, called proper motion. In our vicinity of the galaxy, only a few bright stars exhibit a large enough proper motion to measure over the course of a human lifetime, so their motion does not generally enter into spacecraft navigation. Because of their immense distance, stars can be treated as though they are references fixed in space. (Some stars at the center of our galaxy, though, display tremendous proper motion speeds as they orbit close to the massive black hole located there.)
Nutation
Superimposed on the 26,000-year precession is a small nodding motion with a period of 18.6 years and an amplitude of 9.2 arc seconds. This nutation can trace its cause to the 5 degree difference between the plane of the Moon's orbit, the plane of the Earth's orbit, and the gravitational tug on one other.
Revolution of Earth
Earth revolves in orbit around the Sun in 365 days, 6 hours, 9 minutes with reference to the stars, at a speed ranging from 29.29 to 30.29 km/s. The 6 hours, 9 minutes adds up to about an extra day every fourth year, which is designated a leap year, with the extra day added as February 29th. Earth's orbit is elliptical and reaches its closest approach to the Sun, a perihelion of 147,090,000 km, on about January fourth of each year. Aphelion comes six months later at 152,100,000 km.
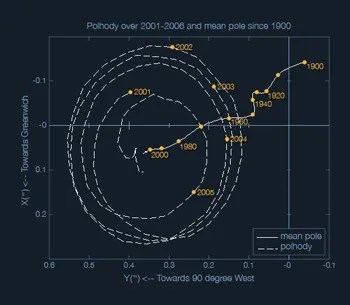
Shorter-term Polar Motion
Aside from the long-term motions, the Earth's rotational axis and poles have two shorter periodic motions. One, called the Chandler wobble, is a free nutation with a period of about 435 days. There is also a yearly circular motion, and a steady drift toward the west caused by fluid motions in the Earth's mantle and on the surface. These motions are tracked by the International Earth Rotation and Reference Systems Service, IERS.
Epochs
Because we make observations from Earth, knowledge of Earth's natural motions is essential. As described above, our planet rotates on its axis daily and revolves around the Sun annually. Its axis precesses and nutates. Even the "fixed" stars move about on their own. Considering all these motions, a useful coordinate system for locating stars, planets, and spacecraft must be pinned to a single snapshot in time. This snapshot is called an epoch.
By convention, the standard reference epoch is J2000.0, which refers to the mean equator and equinox of year 2000, nominally January 1st 12:00 hours Universal Time (UT). The "J" means Julian year, which is 365.25 days long. Only the 26,000-year precession part of the whole precession/nutation effect is considered, defining the mean equator and equinox for the epoch.
The last epoch in use previously was B1950.0 - the mean equator and equinox of 1949, Dec. 31 22:09 UT, the "B" meaning Besselian year, the fictitious solar year introduced by F. W. Bessell in the 19th century. Equations are published for interpreting data based on past and present epochs.
Making Sense
Given an understanding of the Earth's suite of motions -- rotation on axis, precession, nutation, short-term polar motions, and revolution around the Sun -- and given knowledge of an observer's location in latitude and longitude, meaningful observations can be made. This makes it possible to accurately navigate the Solar System. For example, to measure the precise speed of a spacecraft flying to Saturn, you have to know exactly where you are on the Earth's surface as you make the measurement, and then subtract out the Earth's motions from that measurement to obtain the spacecraft's speed. The same applies if you are trying to measure the proper motion of a distant star -- or a star's subtle wobble, to reveal a family of planets.